|
SCALAR RESONANCE
SLIDE 34
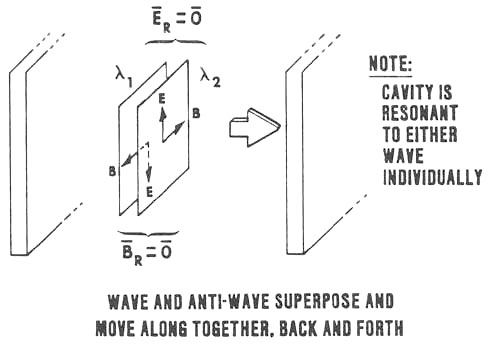
Here we explain a new kind of resonance:
scalar EH resonance, or electrogravitational resonance.
First, imagine we have a standard resonant cavity,
represented by the two walls in our diagram. In this cavity we have a
resonant EM wave moving back and forth, represented by the forward-most
plane. In accordance with convention, we show the E-field vector and the
B-field vector at right angles in this moving wave front. As the wave
front moves back and forth, the vectors vary back and forth; however, at
any one point between the walls, the two vectors always have the same
value. Thus our resonant EM wave forms a standing wave in the cavity.
Now imagine that a second wave front, precisely like
the first and of the same frequency, is superposed over the first wave
front and travels along with it. This second wave -- the
"antiwave" -- has its force vectors 180 degrees out of phase
with the force vectors of
the reference wave. Hence the E-fields and B-fields of
the two superposed waves always sum to vector zeros, anywhere in the
cavity. To an external observer, the cavity contains no ordinary
electromagnetic force fields, hence no ordinary EM energy.
However, the energy density of a single EM sine wave
in vacuum is given by
[E2+B2]/8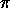 [9]
[9]
This energy density is always positive. Hence the
energy density of the two waves at any point x between the walls is
equal to
[E2+B2]/4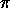 [10]
[10]
where
E
= E(x) ; B =
B(x)
[11]
Thus the energy density of vacuum varies with x. But,
rigorously, since the resultant E and B fields are zero, this describes
a standing gravitational wave. Hence we have a standing EG wave,
existing in the resonant cavity. This is an example of scalar
resonance. Rigorously the cavity has mass and inertia, to an outside
observer, as a result of the two warps in spacetime it contains.
Note that in one half-cycle the energy density of
vacuum is greater than ambient, and in the other half-cycle it is less.
In the region of one half-cycle, time flows at a faster rate than to the
ambient observer, and in the other half-cycle time flows at a slower
rate than to the ambient observer.
One half-cycle appears to contain negative electrical
charge, and the other appears to contain positive charge.
One half-cycle appears to contain a north pole
(positive magnetostatic scalar potential), and the other half-cycle
appears to contain a south pole (negative magnetostatic scalar
potential).
Perhaps now one can begin to understand why a continuously
accelerated orbital electron in the atom does not radiate EM energy,
completely in violation of Maxwell’s equations. The electron is
naught but a complex aspect of a standing scalar resonance, existing
between the nucleus and the orbit.
Next Slide
Previous Slide
Return to Homepage |